Expression for the normal and tangential stresses:

By sing Mohr's circle, derive expression for the normal and tangential stresses on a diagonal plane of material subjected to pure shear. Also state and explain the mohr's theorem for slope and deflection.

Sol.: Mohr circle is graphical method to find out stress system on any inclined plane through body.
It is circle drawn for compound stress system. The centre of circle has the coordinate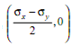   
and radius of circle is

by drawing mohr's circle of stress following 3 systems can be determined
(a) The shear stress, normal stress, and resultant stress on any plane.

(b) Principal stresses and principal planes.

(c) Maximum shearing stresses and the planes of them along with the associated normal stress.

Mohr's circle can be drawn for compound strain system.

1. Mohr's circle of stress with the reference to 2 mutually perpendicular principal stresses acting on the body considers both dike stresses.

Both the principal stresses can be considered as (a) Tensile and (b) Compressive.

Let us for tensile

That is;
 σ_x> σ_y

Steps:

l. Mark OA = S_x and OB =  S_y, along  x-axis (on positive side if tensile and negative side if compressive).

2. Draw circle with BA as diameter, called as mohr's circle of stress.

3. To obtain stress on any plane ¸ as shown in the figure given below measure angle ACP = 2¸ in anti clockwise direction.
4. The normal stress on the plane is S_n = OQ

    Shear stress is τ = PQ

    Resultant stress = σ_r = OP

    And Angle POQ = ? is called as angle of obliquity.

2. Mohr's stress circle for a two dimensional compound stress condition shown in fig.
Let for a tensile
σ_x > σ_y

Steps:

l. Mark OA = σ_x and OB = σ_y along the  x axis

[on positive side if tensile and negative side if compessive.]

2. Mark AC =  τ_xy

and BD =  τ_xy
 
 

 
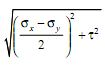
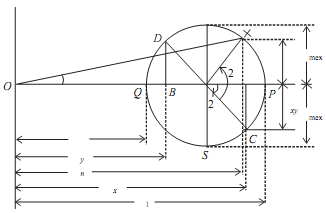
3. Join C and D that bisects AB at E centre of Mohr's circle.

4. With E as centre, EC or ED as radius draw the circle called as Mohr's circle of stress.

5. Point P and Q at which circle cuts S axis gives principal planes OP = σ₁, and OQ = σ₂ gives the 2 principal stresses.

6. ∠CEP = 2θ₁ and ∠CEQ = 2θ₂ can be measured in counter clockwise direction.

θ₁ = (1/2) ∠CEP and θ₁ = (1/2) ∠CEQ indicates principal planes.

7. ER = EC =  τ_max is the maximum shearing stress.

θ′₁ = (1/2) ∠CER and θ′₂

= (1/2) ∠CES

is measured anti-clockwise direction gives maximum shearing planes.

8. To obtain stress on any plane 'θ' measure ∠CEX = 2θ  in anticlockwise direction.

Normal stress on the plane is,

σ_n = OY

Shear Stress, τ = XY

Resultant Stress is σ_r= OX

And

∠XOY = ? is known as angle of obliquity.