=(-d,0,0)+t[(x,y,z)-(-d,0,0)]
=(-d,0,0)+t[x+d,y,z]
=[-d+t.(x+d), t.y, t.z]
Point P' is acquired, while t=t*
There is, P'=(x',y',z') =[-d+t*.(x+d), t*.y, t*.z]
Because, P' lies on X=0 plane shows -d+t*.(x+d)=0 must be true, which is t*=d/(x+d) is actual.
Hence, x'=-d+t*(x+d)=0
y'=t*.y=y.d/(x+d)
z'=t*.z=z.d/(x+d)
Hence P'=( 0, y.d/(z+d), z.d/(x+d))
= (0,y/((z/d)+1), z/((x/d)+1))
In terms of Homogeneous coordinate system as P'=(0,y,z,(x/d)+1). The above equation can become in matrix form that is: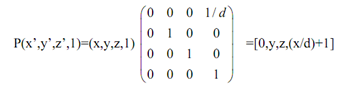
=[0,y/((z/d)+1), z/((x/d)+1),1] -------- (5)
is, P'h = Ph.Pper,x --------------------------------(6)
Here Pper,z in equation (5) implies the particular point perspective transformation w.r.t. x-axis.
Thus, the ordinary coordinates projected point P' of a agreed point P of a particular point perspective transformation with respect to the x-axis as:
(x', y',z',1)= [0,y/((z/d)+1), z/((x/d)+1),1] which has a center of projection at [-d,0,0,1] and a vanishing point assigned on the x-axis at [0,0,0,1]
As the same, the particular point perspective transformation w.r.t. y-axis is consequently:
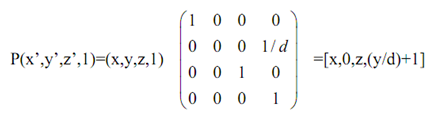
=[x/((y/d)+1),0, z/((y/d)+1),1] That is, P'h = Ph.Pper,y -----------------------------(7)
Here Pper,y in equation (5) implies the particular point perspective transformation w.r.t. y-axis.
Hence, the ordinary coordinates as projected point P' of a given point P of a particular point perspective transformation w.r.t. y-axis that is:
(x',y',z',1)=[x/((y/d)+1),0, z/((y/d)+1),1] which has a center of projection at [0,-d,0,1] and a vanishing point assigned on the y-axis at [0,0,0,1].