Reference no: EM131093873

Question 1. In a one-dimensional problem, consider a particle whose wave function is:

ψ(x) = N(e^ipox/h)/√(x² + a²)

where a and P_o are real constants and N is a normalization coefficient.

a. Determine N so that ψ(x) is normalized.

b. The position of the particle is measured. What is the probability of finding a result between -a/√3 and +a/√3 ?

c. Calculate the mean value of the momentum of a particle which has ψ(x) for its wave function.

Question 2. Consider, in a one-dimensional problem, a particle of mass m whose wave function at time t is ψ/(x, t).

a. At time 1, the distance d of this particle from the origin is measured. Write, as a function of ψ(x, t), the probability p(d_o ) of finding a result greater than a given length d_o. What are the limits of P(d_o) when d_o → 0 and d_o → ∞?

b. Instead of performing the measurement of question a, one measures the velocity v of the particle at time t. Express, as a function of ψ(x, t), the probability of finding a result greater than a given value v_o.

Question 3. The wave function of a free particle, in a one-dimensional problem, is given at time t = 0 by:

ψ(x, 0) - N _-∞∫^+∞dk e^-|k|k_oe^ikx

where k_o and N are constants.

a. What is the probability p(p₁, 0) that a measurement of the momentum, performed at time t = 0, will yield a result included between - p₁ and + p₁? Sketch the function p(p₁, 0).

b. What happens to this probability p(p₁, t) if the measurement is performed at time t? Interpret.

c. What is the form of the wave packet at time t = 0? Calculate for this time the product ΔX.ΔP; what is your conclusion? Describe qualitatively the subsequent evolution of the wave packet.

Question 4. Spreading of a free wave packet

Consider a free particle.

a. Show, applying Ehrenfest's theorem, that < X > is a linear function of time, the mean value < P > remaining constant.

b. Write the equations of motion for the mean values < X² > and (XP + PX>. Integrate these equations.

c. Show that, with a suitable choice of the time origin, the root-mean¬square deviation ΔX is given by:

(ΔX)² = 1/m² (ΔP)₀²t² + (ΔX)₀²

where (ΔX)_o and (ΔP)_o are the root-mean-square deviations at the initial time. How does the width of the wave packet vary as a function of time (see 3-c of complement G₁)? Give a physical interpretation.

Question 5. Particle subject to a constant force

In a one-dimensional problem, consider a particle of potential energy V(X) = - fX, where f is a positive constant [V(X) arises, for example, from a gravity field or a uniform electric field].

a. Write Ehrenfest's theorem for the mean values of the position X and the momentum P of the particle. Integrate these equations; compare with the classical motion.

b. Show that the root-mean-square deviation ΔP does not vary over time.

c. Write the Schrodinger equation in the { |p> } representation. Deduce from it a relation between ∂/∂t|< p|ψ(t)>|² and ∂/∂t|< p|ψ(t)>|². Integrate the equation thus obtained; give a physical interpretation.

Question 6. Consider the three-dimensional wave function

ψ(x, y, z) = Ne-[|x|/2a + |b|/2b + |z|/2x]

where a, b and c are three positive lengths.

a. Calculate the constant N which normalizes ψ.

b. Calculate the probability that a measurement of X will yield a result included between 0 and a.

c. Calculate the probability that simultaneous measurements of Y and Z will yield results included respectively between - b and + b, and - c and + c.

d. Calculate the probability that a measurement of the momentum will yield a result included in the element dp_x, dp_y, dp_z centered at the point p_x = p_y = 0; p_z = h/c.

Question 7. Let ψ (x, y, z) = ψ(r) be the normalized wave function of a particle. Express in terms of ψ(r) the probability for:

a. a measurement of the abscissa X, to yield a result included between x₁ and x₂;

b. a measurement of the component P_x of the momentum, to yield a result included between p₁ and p₂;

c. simultaneous measurements of X and P_z, to yield :

x1 ≤ x ≤ x2

p_z ≥ 0

d. simultaneous measurements of P_x, P_y, P_z, to yield :

P1 ≤ Px ≤ P2

P3 ≤ Py ≤ P4

P5 ≤ Pz ≤ P6

Show that this probability is equal to the result of b when p₃, p₅ → ∞; Pi, P6 ---4" + X ;

e. A measurement of the component U = -1/√3 (X + Y + Z) of the position, to yield a result included between u₁ and u₂.

Question 8. Let J(r) be the probability current associated with a wave function ψ(r) describing the state of a particle of mass m [chap. III, relations (D-17) and (D-19)].

a. Show that:

m ∫d³r J(r) = < P >

where < P) is the mean value of the momentum.

b. Consider the operator L (orbital angular momentum) defined by L = R x P. Are the three components of L Hermitian operators? Establish the relation:

m ∫d³r [r x J(r)] = < L>.

Question 9. One wants to show that the physical state of a (spinless) particle is completely defined by specifying the probability density p(r) = Ψ(r)² and the probability current J(r).

a. Assume the function Ψ(r) known and let ξ(r) be its argument:

Ψ(r) = √ρ(r)^eiξ(r)

Show that :

J(r) = h/mp(r)∇ξ(r)

Deduce that two wave functions leading to the same density p(r) and current J(r) can differ only by a global phase factor.

b. Given arbitrary functions p(r) and J(r), show that a quantum state Ψ(r) can be associated with them only if ∇ x v(r) = 0, where v(r) = J(r)/p(r) is the velocity associated with the probability fluid.

c. Now assume that the particle is submitted to a magnetic field B(r) = ∇ x A(r) [see chap. III, definition (D-20) of the probability current in this case]. Show that:

J = ρ(r)/m [h∇ξ(r) - qA(r)]

and:

∇ x v(r) = -q/m B(r)

Question 10. Virial theorem

a. In a one-dimensional problem, consider a particle with the Hamiltonian:

H = p²/2m + V(X)

where:

V(X) = λXⁿ

Calculate the commutator [H, XP]. If there exists one or several stationary states | φ > in the potential V, show that the mean values < T> and < V> of the kinetic and potential energies in these states satisfy the relation : 2 < T) = n< V>.

b. In a three-dimensional problem, H is written:

H = p²/2m + V(R)

Calculate the commutator [H, R.P]. Assume that V(R) is a homogeneous function of nth order in the variables X, Y, Z. What relation necessarily exists between the mean kinetic energy and the mean potential energy of the particle in a stationary state?

Apply this to a particle moving in the potential V(r) = - e²/r (hydrogen atom).

Recall that a homogeneous function V of n^th degree in the variables x, y and z by definition satifies the relation:

V(αx, αy, αz) = αⁿV(x, y, z)

and satisfies Euler's identity :

x ∂V/∂x + y∂V/∂y + z∂V/∂z = nV(x,y,z).

c. Consider a system of N particles of positions R_i and momenta P_i (i = 1, 2, ... N). When their potential energy is a homogeneous (n^th degree) function of the set of components X_i, Y_i, Z_i, can the results obtained above be generalized?

An application of this can be made to the study of an arbitrary molecule formed of nuclei of charges - Zi_q and electrons of charge q. All these particles interact by pairs through Coulomb forces. In a stationary state of the molecule, what relation exists between the kinetic energy of the system of particles and their energy of mutual interaction?

Question 11. Two-particle wave function

In a one-dimensional problem, consider a system of two particles (1) and (2) with which is associated the wave function ψ (x₁, x₂).

a. What is the probability of finding, in a measurement of the positions X1 and X2 of the two particles, a result such that:

x ≤ x₁ ≤ x + dx

α ≤ x₂ ≤ β

b. What is the probability of finding particle (1) between x and x + dx [when no observations are made on particle (2)] ?

c. Give the probability of finding at least one of the particles between α and β.

d. Give the probability of finding one and only one particle between α and β.

e. What is the probability of finding the momentum of particle (1) included between p₁ and p₂ and the position of particle (2) between α and β.

f. The momenta P₁ and P₂ of the two particles are measured; what is the probability of finding p' ≤ p₁ ≤ p"; p"' ≤ p₂ ≤ p""?

g. The only quantity measured is the momentum P₁ of the first particle. Calculate, first from the results of e and then from those of .1, the probability of finding this momentum included between p' and p". Compare the two results obtained.

h. The algebraic distance X₁ - X₂ between the two particles is measured; what is the probability of finding a result included between - d and + d? What is the mean value of this distance?

Question 12. Infinite one-dimensional well

Consider a particle of mass m submitted to the potential:

V(x) = 0 if 0 ≤ x ≤ a.

V(x)= +∞ if x < 0 or x > a.

| φ_n > are the eigenstates of the Hamiltonian H of the system, and their eigenvalues are E_n = n²Π²h²/2ma² (cf. complement H₁). The state of the particle at the instant t = 0 is :

|Ψ(0) > = a₁ (Ψ₁ > + a₂ |(Ψ₂ > + a₃ l (Ψ₃ > + a₄|(Ψ₄ >

a. What is the probability, when the energy of the particle in the state |Ψ (0)> is measured, of finding a value smaller than 3Π²h²/ma²?

b. What is the mean value and what is the root-mean-square deviation of the energy of the particle in the state |Ψ(t)>?

c. Calculate the state vector |Ψ(t)> at the instant t. Do the results found in a and b at the instant t = 0 remain valid at an arbitrary time t?

d. When the energy is measured, the result 8Π²h²/ma² is found. After the measurement, what is the state of the system? What is the result if the energy is measured again?

Question 13. Infinite two-dimensional well (cf. complement G_II,)

In a two-dimensional problem, consider a particle of mass m; its Hamiltonian H is written :

H  = H_x + H_y

with:

H_x = px²/2m + V(X)    H_y = px²/2m + V(Y)

The potential energy V(x) [or V(y)] is zero when x (or y) is included in the interval [0, a] and is infinite everywhere else.

a. Of the following sets of operators, which form a C.S.C.O. ?

{ H },{H_x}, {H_x, H_y} {H, H_x}

b. Consider a particle whose wave function is:

Ψ(x, y) =  N cos Πx/a.cos Πy/a.sin2Πx/a.sin2Πy/a

when 0 < x < a and 0 < y < a, and is zero everywhere else (where N is a constant).

α. What is the mean value < H > of the energy of the particle? If the energy H is measured, what results can be found, and with what probabilities ?

β. The observable H_x is measured; what results can be found, and with what probabilities? If this measurement yields the result Π²h²/2ma², what will be the results of a subsequent measurement of H_y, and with what probabilities?

γ. Instead of performing the preceding measurements, one now performs a simultaneous measurement of H_x and P_y. What are the probabilities of finding:

E_x = 9Π²h²/2ma²

and :

P_o ≤ P_y ≤ p_o + dp ?

Question 14. Consider a physical system whose state space, which is three-dimensional, is spanned by the orthonormal basis formed by the three kets |u₁ >, |u₂ >, | u₃ >. In this basis, the Hamiltonian operator H of the system and the two observables A and B are written:

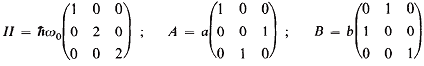
where ω_o, a and b are positive real constants.

The physical system at time t = 0 is in the state:

|Ψ(0) > = 1/√2 |μ₁| > + 1/2 |μ₂> + 1/2|μ₃>

a. At time t = 0, the energy of the system is measured. What values can be found, and with what probabilities? Calculate, for the system in the state 0(0) >, the mean value < H> and the root-mean-square deviation ΔH.

b. Instead of measuring H at time t = 0, one measures A; what results can be found, and with what probabilities? What is the state vector immediately after the measurement?

c. Calculate the state vector |ψ(t)> of the system at time t.

d. Calculate the mean values < A > (t) and < B > (t) of A and B at time t. What comments can be made?

e. What results are obtained if the observable A is measured at time t? Same question for the observable B. Interpret.

Question 15. Interaction picture

(It is recommended that complement F_III and perhaps complement G_III be read before this exercise is undertaken.)

Consider an arbitrary physical system. Denote its Hamiltonian by H_o(t) and the corresponding evolution operator by U_o(t, t'):

Now assume that the system is perturbed in such a way that its Hamiltonian becomes :

H(t) = H_o(t) + W(t)

The state vector of the system in the "interaction picture", |Ψ_I(t)> is defined from the state vector |Ψ_I(t) > in the Schrodinger picture by :

|ψ(t)> = U_o⁺(t, t_o)W(t)U₀(t,t₀)

a. Show that the evolution of I 1P/(t)> is given by :

i^hd/dt|ψI(t)>| = WI(t)|ψ_I(t)>

where W₁(t) is the transform operator of W(t) under the unitary transformation associated with U₀^t(t, t_o)

W₁(t) = U₀^t(t, t_o),W(t) U_o(t, t_o)

Explain qualitatively why, when the perturbation W(t) is much smaller than H_o(t), the motion of the vector |ψs(t)>. is much slower than that of |ψ_s(t)>.

b. Show that the preceding differential equation is equivalent to the integral equation :

|ψ_I(t)> = |ψ_I(t_o)> + 1/ih_to∫^tdt'WI(t')|ψ_I(t'))>

where : |ψ_I(t_o) > = |ψ_s(t)>.

c. Solving this integral equation by iteration, show that the ket |ψ_I(t)> can be expanded in a power series in W of the form:

Question 16. Correlations between two particles

(It is recommended that the complement E₁₁₁ be read in order to answer question e of this exercise.)

Consider a physical system formed by two particles (I) and (2), of the same mass m, which do not interact with each other and which are both placed in an infinite potential well of width a (cf. complement HI, § 2-c). Denote by H(1) and H(2) the Hamiltonians of each of the two particles and by |ψ_I(t)> and |ψ_q(2)> the corresponding eigenstates of the first and second particle, of energies and n²Π²h²/2ma² and n²Π²h²/2ma². In the state space of the global system, the basis chosen is composed of  the states |ψ_nψ_q> defined by:

a. What are the eigenstates and the eigenvalues of the operator H - H(I) + H(2), the total Hamiltonian of the system? Give the degree of degeneracy of the two lowest energy levels.

b. Assume that the system, at time t = 0 is in the state:

|ψ(0)> = 1/√6 |φ1φ1> + 1/√3 |φ1φ2> + 1/√6 |φ2φ1> + 1/√3 |φ2φ2>

a. What is the state of the system at time t?

β. The total energy H is measured. What results can be found, and with what probabilities?

γ. Same questions if, instead of measuring H, one measures H(1).

c. a. Show that |ψ(0)> is a tensor product state. When the system is in this state, calculate the following mean values : < H(1) >, < H(2) > and < H(1)H(2) >.

Compare < H(1) > < H(2) > with < H(1 )H(2) > ; how can this result be explained?

β. Show that the preceding results remain valid when the state of the system is the state |ψ(t)> calculated in b.

d. Now assume that the state |ψ(0) is given by:

|ψ(0> = 1/√5 |φ1φ1> + √(3/5)|φ1φ2> + 1/√5|φ2φ1>

Show that |ψ(0)> cannot be put in the form of a tensor product. Answer for this case all the questions asked in c.

e. a. Write the matrix, in the basis of the vectors |φ1φ2>, which represents the density operator p(0) corresponding to the ket |ψ(0) given in b. What is the density matrix p(t) at time t? Calculate, at the instant t = 0, the partial traces:

p(1) = Tr₂p and p(2) = T_r1p

Do the density operators ρ, ρ(1) and ρ(2) describe pure states? Compare ρ with ρ(1) x ρ(2); what is your interpretation?

β. Answer the same questions as in a, but choosing for |ψ(0)> the ket given in d.

The subject of the following exercises is the density operator: they therefore assume the concepts and results of complement E_III to be known.

Question 17. Let ρ be the density operator of an arbitrary system, where |Χ₁> and Π₁ are the eigenvectors and eigenvalues of p. Write p and p² in terms of the |x₁ and Π₁. What do the matrices representing these two operators in the { |Χ₁> } basis look like - first, in the case where p describes a pure state and then, in the case of a statistical mixture of states?

(Begin by showing that, in a pure case, ρ has only one non-zero diagonal element, equal to 1, while for a statistical mixture, ρ has several diagonal elements included between 0 and 1.) Show that ρ corresponds to a pure case if and only if the trace of ρ² is equal to 1.

Question 18. Consider a system whose density operator is ρ(t), evolving under the influence of a Hamiltonian H(t). Show that the trace of ρ² does not vary over time.

Conclusion : can the system evolve so as to be successively in a pure state and a statistical mixture of states?

Question 19. Let (1) + (2) be a global system, composed of two subsystems (1) and (2). A and B denote two operators acting in the state space ε(1) ⊗ ε(2). Show that the two partial traces Tr₁, { AB } and Tr₁, { BA } are equal when A (or B) actually acts only in the space g(1), that is, when A (or B) can be written :

A = A(1) ⊗ 1(2) [or B = B(1) ⊗ 1(2)].

Application : if the operator H, the Hamiltonian of the global system, is the sum of two operators which act, respectively, only in ε(1) and only in ε(2):

H = H(1) + H(2),

calculate the variation d/dt ρ(1) of the reduced density operator ρ(1). Give the physical interpretation of the result obtained.