Describe about Parallel and Perpendicular Lines ?
Parallel Lines : Parallel lines are coplanar lines (lines that lie in the same plane) that never intersect.

The blue lines are parallel. Notice that these lines have the same slope.
Two lines with the same slope are parallel.
If they had different slope then they would intersect and so they wouldn't be parallel.
If two no vertical lines are parallel, they have the same slope.
What if the lines are vertical?
If two vertical lines are parallel, they both have undefined slope.
(See example 2 for more on horizontal and vertical lines.)
Perpendicular Lines
Perpendicular lines intersect at an angle of 90 degrees, in other words they form a right angle.
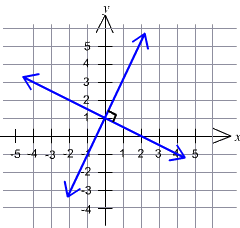
How do the slopes of these perpendicular lines compare to each other?
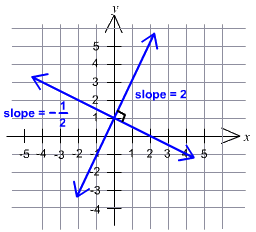
The slopes of the two perpendicular lines are 2 and -1/2. How are these slopes related? -1/2 is the negative reciprocal of 2. (See the basics section for more about negative reciprocals.)
If two lines are perpendicular, their slopes are negative reciprocals.
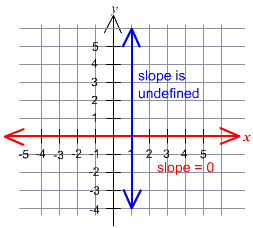
If the slopes of two lines are negative reciprocals, then the lines are perpendicular.
Example 1: What is the slope of a line that is
a. parallel to 12x - 3y = 10?
b. perpendicular to 12x - 3y = 10?
Solution: First let's find the slope of the line 12x - 3y = 10.
Put the equation in the form y = mx + b.
12x - 3y = 10
-3y = -12x +10
-3y/-3 = -12x/-3 +10/-3
Y= -4x -10/3
The slope of the line is -4.
a. All lines that are parallel to 12x - 3y = 10 have slope equal to this line. The slope of a line parallel to 12x - 3y = 10 is -4.
b. All lines that are perpendicular to 12x - 3y = 10 have slope equal to the negative reciprocal of -4. The negative reciprocal of -4 is 1/4, so the slope of a line perpendicular to 12x - 3y = 10 is 1/4.
Example 2: What is the slope of a line that is
a. parallel to the x'axis?
b. perpendicular to the x'axis?
Solution: The x'axis is a horizontal line. All horizontal lines have zero slope.
a. A line parallel to the x'axis has slope = 0.
b. A line perpendicular to the x'axis is vertical. All vertical lines have undefined slope.